Musselman's theorem
In Euclidean geometry, Musselman's theorem is a property of certain circles defined by an arbitrary triangle.
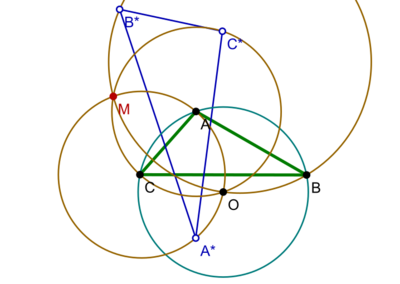
Specifically, let [math]\displaystyle{ T }[/math] be a triangle, and [math]\displaystyle{ A }[/math], [math]\displaystyle{ B }[/math], and [math]\displaystyle{ C }[/math] its vertices. Let [math]\displaystyle{ A^* }[/math], [math]\displaystyle{ B^* }[/math], and [math]\displaystyle{ C^* }[/math] be the vertices of the reflection triangle [math]\displaystyle{ T^* }[/math], obtained by mirroring each vertex of [math]\displaystyle{ T }[/math] across the opposite side.[1] Let [math]\displaystyle{ O }[/math] be the circumcenter of [math]\displaystyle{ T }[/math]. Consider the three circles [math]\displaystyle{ S_A }[/math], [math]\displaystyle{ S_B }[/math], and [math]\displaystyle{ S_C }[/math] defined by the points [math]\displaystyle{ A\,O\,A^* }[/math], [math]\displaystyle{ B\,O\,B^* }[/math], and [math]\displaystyle{ C\,O\,C^* }[/math], respectively. The theorem says that these three Musselman circles meet in a point [math]\displaystyle{ M }[/math], that is the inverse with respect to the circumcenter of [math]\displaystyle{ T }[/math] of the isogonal conjugate or the nine-point center of [math]\displaystyle{ T }[/math].[2]
The common point [math]\displaystyle{ M }[/math] is point [math]\displaystyle{ X_{1157} }[/math] in Clark Kimberling's list of triangle centers.[2][3]
History
The theorem was proposed as an advanced problem by John Rogers Musselman and René Goormaghtigh in 1939,[4] and a proof was presented by them in 1941.[5] A generalization of this result was stated and proved by Goormaghtigh.[6]
Goormaghtigh’s generalization
The generalization of Musselman's theorem by Goormaghtigh does not mention the circles explicitly.
As before, let [math]\displaystyle{ A }[/math], [math]\displaystyle{ B }[/math], and [math]\displaystyle{ C }[/math] be the vertices of a triangle [math]\displaystyle{ T }[/math], and [math]\displaystyle{ O }[/math] its circumcenter. Let [math]\displaystyle{ H }[/math] be the orthocenter of [math]\displaystyle{ T }[/math], that is, the intersection of its three altitude lines. Let [math]\displaystyle{ A' }[/math], [math]\displaystyle{ B' }[/math], and [math]\displaystyle{ C' }[/math] be three points on the segments [math]\displaystyle{ OA }[/math], [math]\displaystyle{ OB }[/math], and [math]\displaystyle{ OC }[/math], such that [math]\displaystyle{ OA'/OA=OB'/OB=OC'/OC = t }[/math]. Consider the three lines [math]\displaystyle{ L_A }[/math], [math]\displaystyle{ L_B }[/math], and [math]\displaystyle{ L_C }[/math], perpendicular to [math]\displaystyle{ OA }[/math], [math]\displaystyle{ OB }[/math], and [math]\displaystyle{ OC }[/math] though the points [math]\displaystyle{ A' }[/math], [math]\displaystyle{ B' }[/math], and [math]\displaystyle{ C' }[/math], respectively. Let [math]\displaystyle{ P_A }[/math], [math]\displaystyle{ P_B }[/math], and [math]\displaystyle{ P_C }[/math] be the intersections of these perpendicular with the lines [math]\displaystyle{ BC }[/math], [math]\displaystyle{ CA }[/math], and [math]\displaystyle{ AB }[/math], respectively.
It had been observed by Joseph Neuberg, in 1884, that the three points [math]\displaystyle{ P_A }[/math], [math]\displaystyle{ P_B }[/math], and [math]\displaystyle{ P_C }[/math] lie on a common line [math]\displaystyle{ R }[/math].[7] Let [math]\displaystyle{ N }[/math] be the projection of the circumcenter [math]\displaystyle{ O }[/math] on the line [math]\displaystyle{ R }[/math], and [math]\displaystyle{ N' }[/math] the point on [math]\displaystyle{ ON }[/math] such that [math]\displaystyle{ ON'/ON = t }[/math]. Goormaghtigh proved that [math]\displaystyle{ N' }[/math] is the inverse with respect to the circumcircle of [math]\displaystyle{ T }[/math] of the isogonal conjugate of the point [math]\displaystyle{ Q }[/math] on the Euler line [math]\displaystyle{ OH }[/math], such that [math]\displaystyle{ QH/QO = 2t }[/math].[8][9]
References
- ↑ D. Grinberg (2003) On the Kosnita Point and the Reflection Triangle. Forum Geometricorum, volume 3, pages 105–111
- ↑ 2.0 2.1 Eric W. Weisstein (), Musselman's theorem. online document, accessed on 2014-10-05.
- ↑ Clark Kimberling (2014), Encyclopedia of Triangle Centers, section X(1157) . Accessed on 2014-10-08
- ↑ John Rogers Musselman and René Goormaghtigh (1939), Advanced Problem 3928. American Mathematical Monthly, volume 46, page 601
- ↑ John Rogers Musselman and René Goormaghtigh (1941), Solution to Advanced Problem 3928. American Mathematics Monthly, volume 48, pages 281–283
- ↑ Jean-Louis Ayme, le point de Kosnitza, page 10. Online document, accessed on 2014-10-05.
- ↑ Joseph Neuberg (1884), Mémoir sur le Tetraèdre. According to Nguyen, Neuberg also states Goormaghtigh's theorem, but incorrectly.
- ↑ Khoa Lu Nguyen (2005), A synthetic proof of Goormaghtigh's generalization of Musselman's theorem. Forum Geometricorum, volume 5, pages 17–20
- ↑ Ion Pătrașcu and Cătălin Barbu (2012), Two new proofs of Goormaghtigh theorem. International Journal of Geometry, volume 1, pages=10–19, ISSN 2247-9880